Direct material yield variance

Direct material yield variance (also known as direct material usage variance) is the result of producing a quantity of output that is different from planned or standard quantity using a certain standard amount of input materials.

A favorable direct material yield variance means a higher production than the standard or expected production based on the standard input quantities of materials. It often results in lower total direct materials cost. An unfavorable variance, on the other hand, means lower production than the standard production based on standard input quantity. Using excessive direct materials than allowed by standards often results in higher total direct materials cost.

Formula

The formula of direct materials yield variance is given below:

Direct material yield variance = (Standard output × Standard cost) – (Actual output × Standard cost)

Or

(Actual quantity used × Standard cost) – (Standard quantity allowed for actual output × Standard cost)

Let’s exemplify the calculation of this variance.

Example

Beta Company processes three materials, namely, material A, material B, and material C, to produce its only product known as product K. This product is produced in powder form and packed into bags before it is shipped to customers and wholesalers.

The three materials are mixed in a standard ratio of 5:4:1 respectively. The standard quantities and costs of materials to produce 1,000 tons of product K are given below:

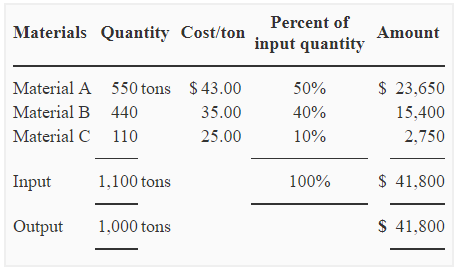
During the month of March, the following quantities of materials were sent to the factory and 32,340 tons of product K was actually produced.

Required: Find the direct material yield variance for Beta Company using above information.

Solution

Computation using output quantities (the first formula):

We can compute material yield variance by comparing standard output quantity and actual output quantity based on standard input quantity. Under this approach, we would use the standard materials cost per unit of output (i.e., $41,800/1,000 tons = $41.80) to convert the quantity variance into dollar variance. Computations are given below:

Direct material yield variance = (Standard output × Standard cost) – (Actual output × Standard cost)
= (*31,000 tons × $41.80**) – (32,340 tons × $41.80)
= $1,295,800 – $1,351,812
= $56,012 Favorable

*34,100 × (1,000/1,100)
**$41,800/1,000 tons = $41.80

The material yield variance for March was favorable because company actually produced 32,340 tons of output which was higher than the standard output of 31,000 tons based on input quantity of 34,100 tons.

Computation using input quantities (the second formula):

The computation of above variance is also possible by using input quantities of materials. Under this approach we would compare actual quantity of materials used and standard quantity of materials allowed for actual output. To convert the quantities into dollars, we would use the weighted average of standard materials cost (i.e., $41,800/1,100 tons = $38.00). Computations are given below:

Direct material yield variance = (Actual quantity used × Standard cost) – (Standard quantity allowed for actual output × Standard cost)
= (34,100 tons × $38*) – (**35,574 tons × $38)
= 1,295,800 – 1,351,812
= $56,012 Favorable

*$41,800/1,100 tons = $38.00
**32,340 × (1,100/1,000) = 35,574 tons

According to standards, the company was allowed to use an input of 35,574 tons to produce an output of 32,340 tons (the actual output). However, it used only 34,100 tons of materials which resulted in a favorable direct material yield variance.

Practice exercises and problems
Practice quizzes